Application of two heavy mineral analysis methods in the provenance study of Irrawaddy River sediments on the southeastern margin of Tibetan Plateau
-
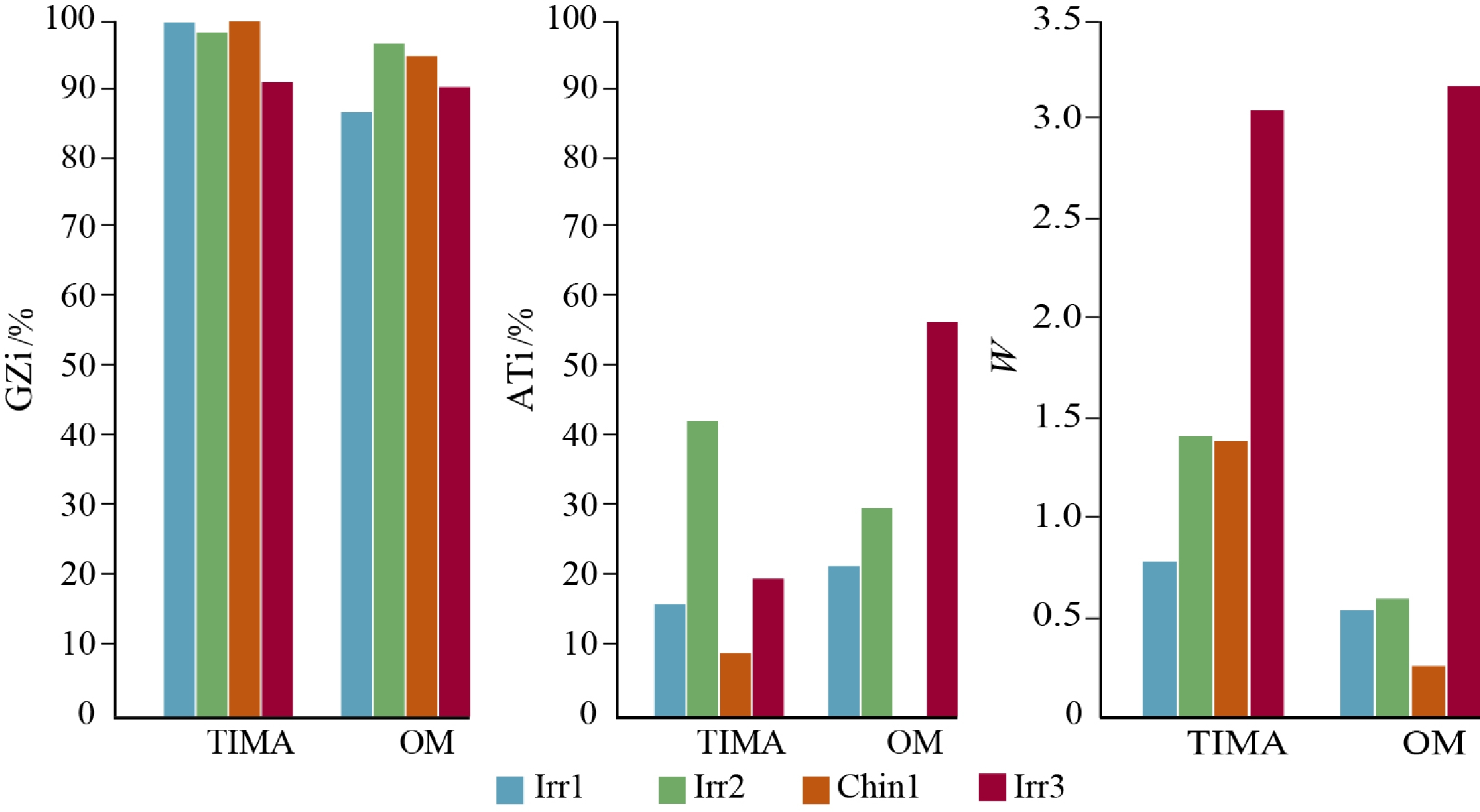
摘要: 伊洛瓦底江是亚洲大型河流之一，其沉积物记录了青藏高原周缘造山带的剥蚀风化信息，对该流域沉积物的溯源研究是探究高原隆升对水系演化影响的重要课题。沉积物的重矿物种类与源岩联系紧密，是物源分析的重要手段之一。重矿物鉴定分析手段层出不穷，但不同鉴定手段之间缺乏对比分析。采用自动矿物分析系统TIMA与光学显微镜鉴定(optical microscope, OM)两种方法对伊洛瓦底江沉积物重矿物进行了鉴定分析，结果表明：伊洛瓦底江上游主要重矿物是角闪石-石榴子石-赤/磁铁矿、钛铁矿；伊洛瓦底江下游主要重矿物是赤/磁铁矿、钛铁矿-石榴子石-黝帘石-角闪石；支流钦敦江主要重矿物组合是黝帘石-角闪石-石榴子石。两种方法的结果都指示了伊洛瓦底江沉积物主要来自上游流经的缅甸北部构造单元的变质岩以及中/基性岩，太公-密支那带对伊洛瓦底江沉积物贡献量最大，钦敦江流域对伊洛瓦底江下游沉积物贡献量有限。但是两种方法对重矿物种类以及单个重矿物含量鉴定结果有着明显差异：TIMA方法的鉴定种类更加丰富，且其分析结果与伊洛瓦底江流域地质岩性分布的耦合程度更高，但TIMA无法区分化学性质相同的矿物；OM法对光学性质相近的矿物鉴定结果不准确。因此建议对重矿物分类程度或精确性要求更高的研究使用TIMA进行精确分析，同时辅助OM法区分化学性质相同的矿物。Abstract: The Irrawaddy River is one of the large rivers in Asia, and its sediments record the denudation and weathering information of the orogenic belt around the Tibetan Plateau. Tracing the sediments in this basin is an important topic to explore the impact of plateau uplift on the evolution of river system. The types of heavy minerals in sediments are closely related to source rocks, which is one of the important means for provenance analysis. There are a variety of methods to identify and analyze heavy minerals, but there is a lack of comparative analysis among different methods. This paper adopted TESCAN Integrated Mineral Analyzer (TIMA) and Optical Microscope (OM) to identify and analyze heavy minerals of Irrawaddy River sediments. The main heavy minerals in the upper reaches of the Irrawaddy are amphibole-garnet- hematite/magnetite, ilmenite. The main heavy minerals in the lower reaches of the Irrawaddy are hematite/magnetite, ilmenite-garnet-zoisite-hornblende. The main heavy mineral combination of the tributary Chindwin River is zoisite-hornblende-garnet. The results of both methods indicate that the Irrawaddy sediments are mainly derived from metamorphic and intermediate or mafic rocks of tectonic units in northern Myanmar, with the Tagaung-Myitkyina Belt contributing the most to the Irrawaddy sediments and the Chindwin River basin contributing a limited amount to the lower reaches of the Irrawaddy sediments. However, there are significant differences in the identification results of heavy mineral species and individual heavy mineral contents between the two methods. The identification species of TIMA method are more abundant, and its analysis results are more coupled with the geological lithological distribution of Irrawaddy River basin. It’s also found that TIMA cannot distinguish the minerals with the same chemical properties, and OM method is not accurate in identifying the minerals with similar optical properties. Therefore, it is promoted in this paper that TIMA should be used for accurate analysis in studies requiring more precise and accurate classification of heavy minerals, while the OM method should be supplemented to distinguish minerals with the same chemical properties.
-
Keywords:
- TIMA /
- optical microscope /
- heavy minerals source analysis /
- Irrawaddy River /
- Tibetan Plateau
-
1. 研究背景
S变换[1]是由美国的地球物理学家Stockwell等[2-3]在短时傅里叶变换和小波变换的基础上提出的。与短时傅里叶变换和小波变换相比，S变换既解决了短时傅里叶变换时窗固定的问题，同时具有小波变换的多分辨率分析。由于S变换所具有的独特优势，其在信号分析和处理领域受到广泛关注。由于高斯时窗和频率的函数关系较为单一，降低了S变换在实际应用中的灵活性，因此很多学者从不同角度对S变换进行了推广和改进得到广义S变换[4-8]，使得广义S变换更加适用于地震资料处理、油气检测[9-11]等方面的信号处理。
广义S变换作为时频域的滤波工具，能够压制噪音从而提高地震资料信噪比[12]。赵淑红等[13]首先在频域和时域确定干扰波范围，再利用广义S变换处理VSP数据；李雪英等[14]考虑到地震信号有效频带随时间逐渐变窄，在时频域采用交互式的方法确定高频噪音切除边界；王云专等采用时频谱叠加的方法计算共中心点道集中的滤波器进而压制随机干扰[15]。陈学华等引入λ和p两个参数控制高斯窗函数的变化趋势，在应用过程中通过试验确定最优参数[5]。本文对陈学华[5]的广义S变换方法进行了改进，使其兼顾对高低频端信号的分析能力，能够更加适用于随机噪音压制；其次由于标准S逆变换存在能量泄露，通过修正S逆变换[16-19]，以消除滤波噪音。在压制噪音时，首先计算信号瞬时信噪比，根据瞬时信噪比的高低结合不同处理策略实现噪音压制。通过处理合成数据和实际资料，验证改进广义S正反变换在随机噪音压制方面的有效性。
2. 方法原理
2.1 改进广义S变换
信号u(t)的标准S正变换的表达式为
$$ \int S\left(\tau ,f\right)={\int }_{-\infty }^{\infty }u\left(t\right)w(\tau -t,f){\mathrm{e}}^{-i2{{\text π} }ft}\mathrm{d}t $$ (1) 式中，
$ S\left(\tau ,f\right) $ 是信号$ u\left(t\right) $ 的时频谱，$ w(t,f) $ 是高斯窗函数，其具体表达式为$$ w\left(t,f\right)=\frac{1}{\sigma \sqrt{2\mathrm{{\text π} }}}{\mathrm{e}}^{\frac{-{t}^{2}}{2{\sigma }^{2}}} $$ (2) 式中，
$ \sigma =\dfrac{1}{\left|f\right|} $ 是控制高斯窗宽度的尺度因子。广义S变换就是通过调节尺度因子大小控制对应的高斯窗函数的有效宽度。针对标准S变换高斯窗形态随频率变化趋势单一进而导致S变换时频谱聚焦度低的问题，陈学华等[5]在标准S变换的基础上引入了控制参数λ和p，目的为丰富尺度因子随频率的变化趋势，针对不同的资料提供更多的参数组合。其改进的尺度因子为
$$ \sigma =\frac{1}{\lambda {\left|f\right|}^{p}} $$ (3) 不同的控制参数λ和p影响尺度因子变化的快慢（图1）。不同的λ值对尺度因子的主要影响集中在低频端，即随着λ值的增大，同一频率对应的尺度因子幅值增大。不同的p值对尺度因子的主要影响在高频端，即随着p值的增大，同一频率对应的尺度因子幅值减小。为了进一步说明两个参数对时变信号的聚焦作用，选取理论信号进行效果分析。该信号是由两个不同的宽频带调频信号组成，即使得高斯窗尺度因子随频率的变化范围比较大，可以有效检验方法的适用性。
![]()
图 1 理想时频谱与不同控制参数的广义S变换的时频谱a. 理想时频；b. p=0.7，λ=1对应的时频谱；c. p=0.9, λ=1对应的时频谱；d. p=1，λ=1标准S变换时频谱；e. p=1.2，λ=1对应的时频谱；f. p=1，λ=1.2对应的时频谱；g. p=1，λ=0.9对应的时频谱；h. p=1，λ=0.6对应的时频谱。Figure 1. Ideal time-frequency spectrum and time-frequency spectrum of S transform using different control factorsa. ideal time-frequency spectrum, b. p=0.7, λ=1 corresponding to time-frequency spectrum, c. p=0.9, λ=1 corresponding to time-frequency spectrum, d. p=1, λ=1 corresponding to time-frequency spectrum, e. p=1.2, λ=1 corresponding to time-frequency spectrum, f. p=1, λ=1.2 corresponding to time-frequency spectrum, g. p=1, λ=0.9 corresponding to time-frequency spectrum, h. p=1, λ=0.6 corresponding to time-frequency spectrum.$$\begin{split} y=&{\sin}\left\{3*\left[140{{\text π} }t+40{\sin}\left(3{{\text π} }t\right)\right]\right\}+\\&{\sin}\left\{3*\left[140{{\text π} }t+40{\cos}\left(3{{\text π} }t+120\right)\right]\right\} \end{split}$$ (4) 采用不同控制参数λ和p对上述信号进行广义S变换，分析对应的时频谱聚焦程度。当p值由小变大时，信号时频谱在低频端与高频端的聚焦程度均响应明显。其中低频端的聚焦程度随p值增大而变好，高频端随p值增大而变差，甚至当p＞1时，高频端出现严重失焦（图1a-d）。当p值由小变大时，信号时频谱在低频端的聚焦程度无响应，而高频端的聚焦程度也变化不大（图1c、f、g、h）。广义S变换中参数λ和p只能取固定值，其单一性的变化趋势不能兼顾高低频端的信号聚焦程度。综合以上实验结果可知，p值对时频域聚焦程度影响更大。改进广义S变换主要是更改p值变化趋势，而固定λ=1。
p值由小变大时，低频端与高频端聚焦程度响应不同。高频端信号需要小的p值，而低频端信号需要大的p值。为实现广义S变换在时频域的宽频信号扫描，将参数p改为频率的线性函数，即
$$ p=p\left(f\right)=a+bf $$ (5) 其中，初始值a＞0，变化率b＜0。
在S变换计算过程中，计算的最小频率为0 Hz，最大频率为待分析信号的Nyquist频率，在这个频带范围内规定p值线性递减，同时调整p值的初始值a和变化率b，使p值能够自动匹配信号，增加信号时频分析的聚焦程度。
为了检验该方法的效果，以理论模拟信号为例进行时频分析对比。该信号是由两个频率变化趋势相反的线性调频信号和一个正弦调频信号组成，见公式（6）。图2是标准S变换、广义S变换的优选方案（p=0.8，λ=1）和改进广义S变换的时频谱图（p=0→1，λ=1）。由图2可知，标准S变换在频率方向出现了条带状能量团，模糊了真实时频谱。广义S变换改善了时频谱图，增强了时频谱的聚焦度，而改进的广义S变换其高频的线性调频信号时频谱聚焦度更高，正弦型调频信号能量分布更均匀，分析效果要优于广义S变换。
![]()
图 2 合成信号时频谱图a. 标准S变换，b. 广义S变换（p=0.8，λ=1），c. 改进的广义S变换（p=0→1，λ=1）。Figure 2. Synthetic signal time-frequency spectruma. the standard S transform, b. conventional generalized S transform (p=0.8, λ=1), c. improved generalized S transform (p=0→1, λ=1).$$\begin{split} y=&{\cos}\left(68{{\text π} }t-20{{\text π} }{t}^{2}\right)+{\cos}\left[2{{\text π} }{\sin}\left(5{{\text π} }t\right)+120{{\text π} }t\right]+\\&{\cos}(168{{\text π} }t+28{{\text π} }{t}^{2}) \end{split}$$ (6) 为了进一步检验改进的广义S变换在地震信号分析中的适用性，选取一道添加高斯白噪声的实际地震信号进行分析。在高频噪声存在的情况下，利用上述三种方法得到时频谱的能量分布（图3）。由图3中立体图与平面图对比可知，标准S变换对低频部分的信号分析结果聚焦度比较好，但其高频噪音的时频谱的能量被过分放大，如果用这种方法在时频域采用阈值滤波的方法进行去噪，显然会把部分信号当成噪音同时也无法把高频噪音去掉。广义S变换的时频谱改善了高频噪音部分的聚焦度，但在低频端能量团几乎连在一起，时间分辨率变差。改进的广义S变换和标准S变换在低频端的能量分布相近，在高频端使高频噪音的能量分散聚焦，而且各个时频点的能量比较稳定，没有被过分放大。可以看出改进的广义S变换对地震资料进行分析或者时频域滤波，效果显然要优。
![]()
图 3 含随机噪声的地震信号时频谱图a. 标准S变换，b. 广义S变换（p=0.8, λ=1），c. 改进的广义S变换（p=0→1, λ=1）。Figure 3. Time-frequency spectrum of seismic signal with random noisea. the standard S transform, b. conventional generalized S transform (p=0.8, λ=1), c. improved generalized S transform (p=0→1, λ=1) .2.2 改进S域时频滤波
由于广义S变换具有逆变换，因此，可以利用广义S正反变换实现时频滤波。其常规滤波思路是利用二维滤波函数F(τ，f )与S变换时频谱相乘，对乘积结果沿时间轴积分，再反傅氏变换回时间域。滤波公式为：
$$ {u}_{\mathrm{f}\mathrm{i}\mathrm{l}\mathrm{t}1}\left(t\right)={\int }_{-\mathrm{\infty }}^{\mathrm{\infty }}{\int }_{-\mathrm{\infty }}^{\mathrm{\infty }}{S}(\tau ,f){F}(\tau ,f){\mathrm{e}}^{i2\mathrm{{\text π} }ft}\mathrm{d}\tau \mathrm{d}f $$ (7) 式中，F(τ, f )是时频域滤波函数。通过计算发现当F(τ, f ) =1时，即不对时频谱作任何处理可以完全重构信号，但当F(τ, f ) ≠1时，实际滤波结果和理想滤波结果存在差异，无法保证时频滤波的准确性[10]，如图4所示，在时频域将合成信号为500～600 ms的时频谱滤掉，反变换回时间域就出现了能量泄露。
![]()
图 4 标准S逆变换时频滤波效果图a. 合成信号，b. 合成信号时频域滤波，c. 时间域滤波效果。Figure 4. Effect of time and frequency filtering of standard S inverse transforma. synthetic signal, b. time and frequency filtering of synthetic signal, c. filtering effect of time field.为了解决这个问题，需要修正变换公式得到精确滤波的改进S域时频滤波公式。
$$\begin{split} S\left(\tau ,f\right)=&{\int }_{-\infty }^{\infty }u\left(t\right)w\left(\tau -t,f\right){\mathrm{e}}^{-i2\mathrm{{\text π} }ft}\mathrm{d}t=\\&{\int }_{-\infty }^{\infty }u\left(t\right)\frac{\lambda {\left|f\right|}^{p}}{\sqrt{2\mathrm{{\text π} }}}{\mathrm{e}}^{\frac{-{\lambda }^{2}{f}^{2p}{(\tau -t)}^{2}}{2}}{\mathrm{e}}^{-i2\mathrm{{\text π} }ft}\mathrm{d}t\end{split}$$ (8) 式中，u(t)是待分析信号，w(τ-t, f)是窗函数。
根据高斯窗函数的表达式，引入一个新的变量x(τ, t)。
$$ x\left(\tau ,t\right)=u\left(t\right){\mathrm{e}}^{\frac{-{\lambda }^{2}{f}^{2p}{(\tau -t)}^{2}}{2}} $$ 对式（8）针对变量t做傅里叶变换：
$$ x\left(\tau ,f\right)={\int }_{-\infty }^{\infty }u\left(t\right){{\mathrm{e}}^{\frac{-{\lambda }^{2}{f}^{2p}{(\tau -t)}^{2}}{2}}\mathrm{e}}^{-i2\mathrm{{\text π} }ft}\mathrm{d}t $$ (9) 对比式（7）和式（9）可得
$$ x\left(\tau ,f\right)=\frac{\sqrt{2\mathrm{{\text π} }}}{\lambda {\left|f\right|}^{p}}S\left(\tau ,f\right) $$ (10) 对式（10）针对变量 f 作反傅里叶变换：
$$ x\left(\tau ,t\right)=\frac{\sqrt{2\mathrm{{\text π} }}}{\lambda }{\int }_{-\infty }^{\infty }\frac{S\left(\tau ,f\right)}{{\left|f\right|}^{p}}{\mathrm{e}}^{i2\mathrm{{\text π} }ft}\mathrm{d}f $$ (11) 由式（8）可知，当τ=t时，u(t)和x(τ, t)之间具有对应关系：
$$ u\left(t\right)=x(t,t) $$ (12) 因此，令τ=t代入式（11）可得：
$$ u\left(t\right)=x\left(t,t\right)=\frac{\sqrt{2\mathrm{{\text π} }}}{\lambda }{\int }_{-\infty }^{\infty }\frac{S\left(t,f\right)}{{\left|f\right|}^{p}}{\mathrm{e}}^{i2\mathrm{{\text π} }ft}\mathrm{d}f $$ (13) 对u(t)进一步展开：
$$ \begin{split}u\left(t\right)=&\frac{\sqrt{2\mathrm{{\text π} }}}{\lambda }{\int }_{-\infty }^{\infty }\frac{S\left(t,f\right)}{{\left|f\right|}^{p}}{\mathrm{e}}^{i2\mathrm{{\text π} }ft}\mathrm{d}f=\\&{\int }_{-\infty }^{\infty }u\left(t\right){\mathrm{e}}^{\frac{-{\lambda }^{2}{f}^{2p}{\left(\tau -t\right)}^{2}}{2}}{\mathrm{e}}^{-i2\mathrm{{\text π} }ft}\mathrm{d}t=\\&{\int }_{-\infty }^{\infty }u\left(\tau \right){\int }_{-\infty }^{\infty }{\mathrm{e}}^{\frac{-{\lambda }^{2}{f}^{2p}{\left(t-\tau \right)}^{2}}{2}}{\mathrm{e}}^{-i2\mathrm{{\text π} }f(t-\tau )}\mathrm{d}f\mathrm{d}\tau =\\&{\int }_{-\infty }^{\infty }u\left(\tau \right)m\left(t-\tau \right)\mathrm{d}\tau =u\left(t\right)\otimes m\left(t\right)\end{split} $$ (14) 式中，
$ \otimes $ 代表褶积，函数m(t)的表达式：$$ m\left(t\right)={\int }_{-\infty }^{\infty }{\mathrm{e}}^{\frac{-{\lambda }^{2}{f}^{2p}{t}^{2}}{2}}{\mathrm{e}}^{i2\mathrm{{\text π} }ft}\mathrm{d}f $$ (15) 由式（14）可知，计算得到的结果是原始信号和信号m(t)的褶积，因此在时频域滤波结果的基础上需要再做一步滤波修正处理，最终的滤波表达式为：
$$ {u}_{filt2}\left(t\right)=\frac{\sqrt{2\mathrm{{\text π} }}}{\lambda }{\int }_{-\infty }^{\infty }\frac{S(t,f)F(t,f)}{{\left|f\right|}^{p}}{\mathrm{e}}^{i2\mathrm{{\text π} }ft}\mathrm{d}f $$ (16) $$ {u}_{\mathrm{f}\mathrm{i}\mathrm{l}\mathrm{t}3}\left(t\right)={\rm{IFT}}\left(\frac{{U}_{\mathrm{f}\mathrm{i}\mathrm{l}\mathrm{t}2}\left(f\right)}{M\left(f\right)}\right) $$ (17) 式中，F(t, f)是时频域滤波函数，Ufilt2(f)是ufilt2(t)的频谱，M(f)是m(t)的频谱，IFT表示反傅里叶变换。式（16）和式（17）是最终的滤波公式。
为了检验对S逆变换的改进效果，分别用标准逆变换、改进逆变换将图4b中的时频谱逆变换，并计算与准确滤波结果的差值，如图5所示。对比结果显示，改进逆变换消除了滤波区信号的畸变，避免产生滤波噪音。
![]()
图 5 准确滤波结果和不同滤波方法计算的差值a. 准确滤波结果，b. 标准逆变换计算差值（红色）和改进逆变换（蓝色）计算差值。Figure 5. Accurate filtering result and difference calculated by different filtering methodsa. accurate filtering result, b. difference calculated by standard S inverse transform (red) and difference calculated by improved S inverse transform (blue).基于上述改进广义S变换和修正的S逆变换，可以将随机噪音压制概括为3步：① 对含噪信号进行改进广义S变换得到信号的S谱；② 在S谱的基础上利用谱比法[20]计算信号的瞬时信噪比；③ 根据瞬时信噪比的不同，采用指数衰减和阈值滤波两种策略压制随机噪音。具体处理步骤如下：
（1）计算信号的S变换时频谱，利用该时频谱计算瞬时信噪比和阈值。阈值的计算思路是：计算初至前两个频率段内噪音的时频谱的平均值，作为初始阈值，具体的频率段可以分成有效频带内和有效频带外两段，计算两个阈值。由于高频随机干扰的时频谱幅值的均值随时间变化不大，而相对浅层数据深层数据的高频成分衰减快，能量弱，高频噪音能量占优，因此在无法利用初至前记录计算阈值时也可利用深层数据较高频段的时频谱计算初始阈值。
$$ {E}_{\mathrm{m}\mathrm{e}\mathrm{a}\mathrm{n}}\left(t,f\right)=\frac{1}{T\times F}\sum _{t={t}_{1}}^{{t}_{2}}\sum _{f={f}_{1}}^{{f}_{2}}\left|S(t,f)\right| $$ (18) 式中，Emean(t, f)是阈值，S(t, f)是时频谱，T是参与计算时间点个数，F是参与计算频率点个数，t1到t2是参与计算的时间段，f1到f2是参与计算的频率段。
（2）实际地震数据需要考虑地层的吸收衰减，且高频衰减更快，因此深部有效频带变窄，有效信号能量弱[21]，此时如果将深部地震记录按照阈值处理，会损失很多有效信号，因此为了保留一部分有效信号以供后续处理和进一步使用，此时对瞬时信噪比低的数据段采用指数衰减的方法处理，即对信噪比小于1的时间点，对该时间点大于有效频带高频端的S谱的幅值进行一个指数衰减的加权，即将S(t, f)乘以一个以f为变量的指数衰减函数H(f)，如式（19）：
$$ H\left(f\right)={\mathrm{e}}^{-\frac{f}{\mu }} $$ (19) 式中，μ是一个衰减系数，f是频率，μ越大对高频衰减越小，反之对高频衰减越大。
（3）当瞬时信噪比大于1时，有效信号能量占优，且随机噪音的时频谱分布在整个频带上，对该时间点所有S谱采用阈值滤波法，当某频率点的幅值小于r倍的Emean(t, f)的时候，定义该点的S谱为噪音，将其充零，当其幅值大于r倍的Emean(t, f)的时候，将其时频谱的幅值减去r倍的Emean(t, f)，并将处理之后的时频谱反变换回时间域。
在实际应用中，应根据实际地震资料的情况，进行反复试验，选取合适的μ和r值，既能压制随机噪音，又能保护高频端的有效信号，为数据拓频留有空间。
3. 合成信号试验
为了检验方法的有效性，首先利用零相位雷克子波模拟48道经地层吸收衰减的地震记录，加入随机白噪声后，利用改进广义S变换对地震记录压制随机噪音（图6）。抽取单道地震模拟数据对比，能够清晰地看出改进广义S变换压制随机噪声的效果，尤其深部低信噪比信号也得到有效的恢复（图7）。分析时频域中滤波效果，可看到有效信号时频谱得以保留，随机噪音时频谱得到有效压制（图8）。
![]()
图 6 含噪地震记录去噪效果图a. 48道模拟地震记录， b. 含白噪声的模拟地震记录， c. 去除噪声的模拟地震记录。Figure 6. Denoising effect for seismic records with noisea. 48 channels simulated seismic record, b. simulated seismic record with white noise, c. simulated seismic record after denoising.![]()
图 7 单道信号对比图a. 加白噪声后与未加噪声单道信号对比， b. 去噪后与未加噪声单道信号对比。Figure 7. Comparison of single channel signalsa. comparison of single channel signals with noise and those without noise, b. comparison of single channel signals without noise and those after noise reduction.![]()
图 8 时频域滤波效果对比a. 不含白噪声模拟地震道的时频谱，b. 含白噪声模拟地震道的时频谱，c. 去除噪声模拟地震道的时频谱。Figure 8. Comparison of filtering effects in time-frequency domaina. time and frequency spectrum of simulated seismic channel without noise, b. time and frequency spectrum of simulated seismic channel with white noise, c. time and frequency spectrum of simulated seismic channel after noise reduction.4. 实际资料处理
为了检验本文改进的广义S变换方法在实际资料中的应用效果，选择高分辨率海洋二维地震叠前剖面进行去噪处理，并将其与传统广义S变换滤波去噪法进行对比。本文首先通过单炮初至前的噪声信号确定去噪阈值，再利用改进的广义S变换进行时频滤波。图9为地震叠前单炮剖面。传统广义S变换参数经过对比后确定为（p=0.6, λ=1），改进后的广义S变换应用参数（p=0.6→1, λ=1）。图10是两种方法压制噪声效果图。从整体上可以看出，两种广义S变换时频域去噪均能达到一定效果，随机噪声得到合理去除。但改进的广义S变换在远偏移距处的弱信号去噪效果更好，深部反射同相轴连续性更优，有效信号的损失较小，对弱信号起到保护作用。
![]()
图 10 两种方法压制噪声效果对比a. 改进广义S变换压噪后剖面，b. 传统广义S变换压噪后剖面，c. 改进广义S变换去除的噪声，d. 传统广义S变换去除的噪声。Figure 10. Comparison of noise suppression effects between two methodsa. denoised profile by improved generalized S transform, b. denoised profile by traditional generalized S transform, c. noise removal by improved generalized S transform, d. noise removal by generalized S transform.5. 结论
本文针对广义S变换高斯窗参数λ和p固定、逆变换存在信号泄露的问题，提出了改进的思路和计算过程，并将其应用于随机噪音压制。通过对合成信号和实际资料的应用，得到以下认识：
（1）改进广义S变换时频谱聚焦度要优于广义S变换，而且参数p与频率f呈线性变化，以适应不同的应用目的；改进后的逆变换可以实现精确滤波，消除了滤波噪音。
（2）利用单炮初至前或深部较高频段时频谱计算阈值，根据每个时刻时频谱的幅值与阈值的差异压制噪音，同时考虑到地震信号的有效频带和瞬时信噪比随时间变化的情况，对瞬时信噪比较低的采用指数衰减的策略压制噪音，可以在保护有效信号的基础上实现去噪目的。
（3）高斯窗参数
$ p $ 的范围选择，应当根据高低频端的聚焦程度而改变。通过试验确定的参数$ p $ ，既能够有效压制噪音又能避免弱有效信号的损失，为后续处理打好基础。 -
![]()
图 1 伊洛瓦底江流域地形以及主要构造单元分布图
A：伊洛瓦底江流域地形图，B：伊洛瓦底江流域主要构造单元分布图（据Mitchell, 2007[28]和缅甸1∶100万地质图）。
Figure 1. The topography and the distribution of major tectonic units in Irrawaddy River Basin
A: The topography in Irrawaddy River Basin, B: the distribution of major tectonic units in Irrawaddy River Basin.
![]()
图 2 TIMA自动扫描获得的伊洛瓦底江沉积物重矿物图像
Figure 2. Automatic scanning images of heavy minerals in Irrawaddy River sediments by TIMA
![]()
图 3 伊洛瓦底江沉积物重矿物组合特征
Figure 3. The characteristics of heavy mineral assemblages of Irrawaddy River sediments
![]()
图 4 伊洛瓦底江沉积物重矿物特征指数柱状图
Figure 4. Histogram of characteristic index of heavy minerals in Irrawaddy River sediments
![]()
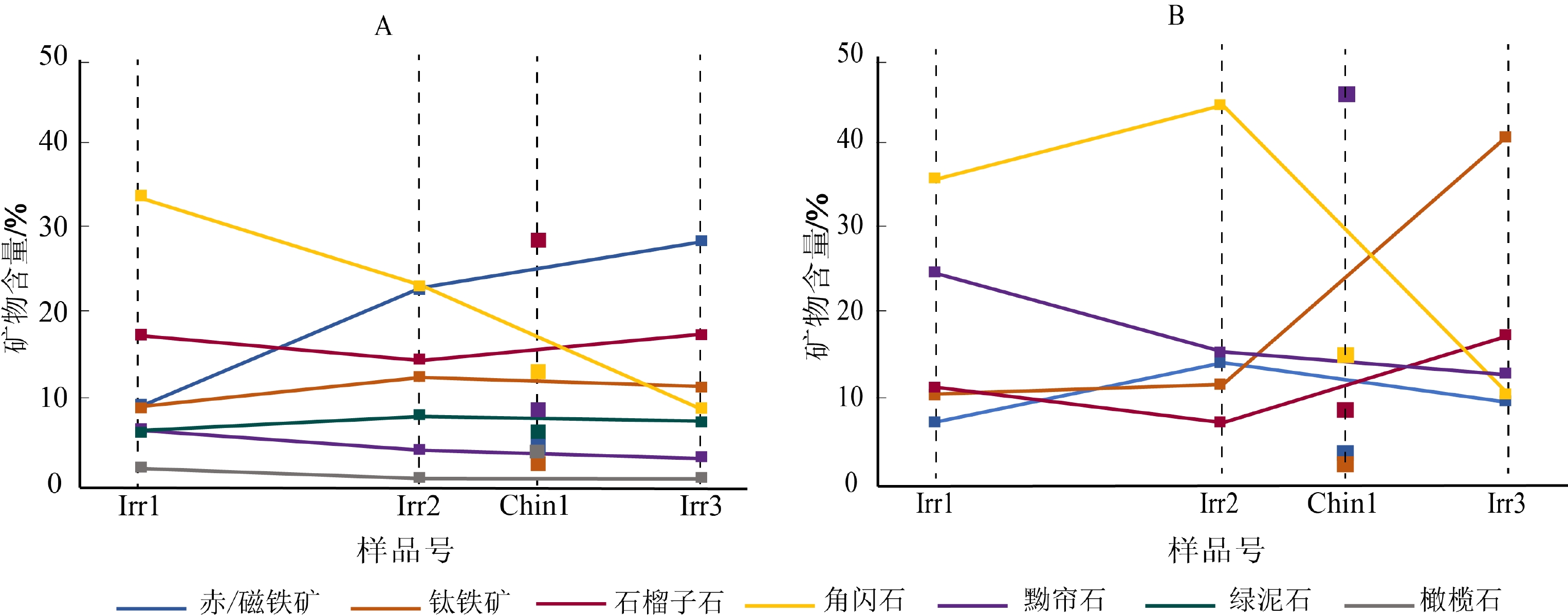
图 5 伊洛瓦底江沉积物主要重矿物含量变化折线图
A：TIMA测得伊洛瓦底江主要重矿物变化，B：OM法测得伊洛瓦底江主要重矿物变化。
Figure 5. The line chart of changes in heavy mineral content of Irrawaddy River sediments
A: The major heavy mineral changes of Irrawaddy River sediments by TIMA, B: The major heavy minerals changes of Irrawaddy River sediments by OM.
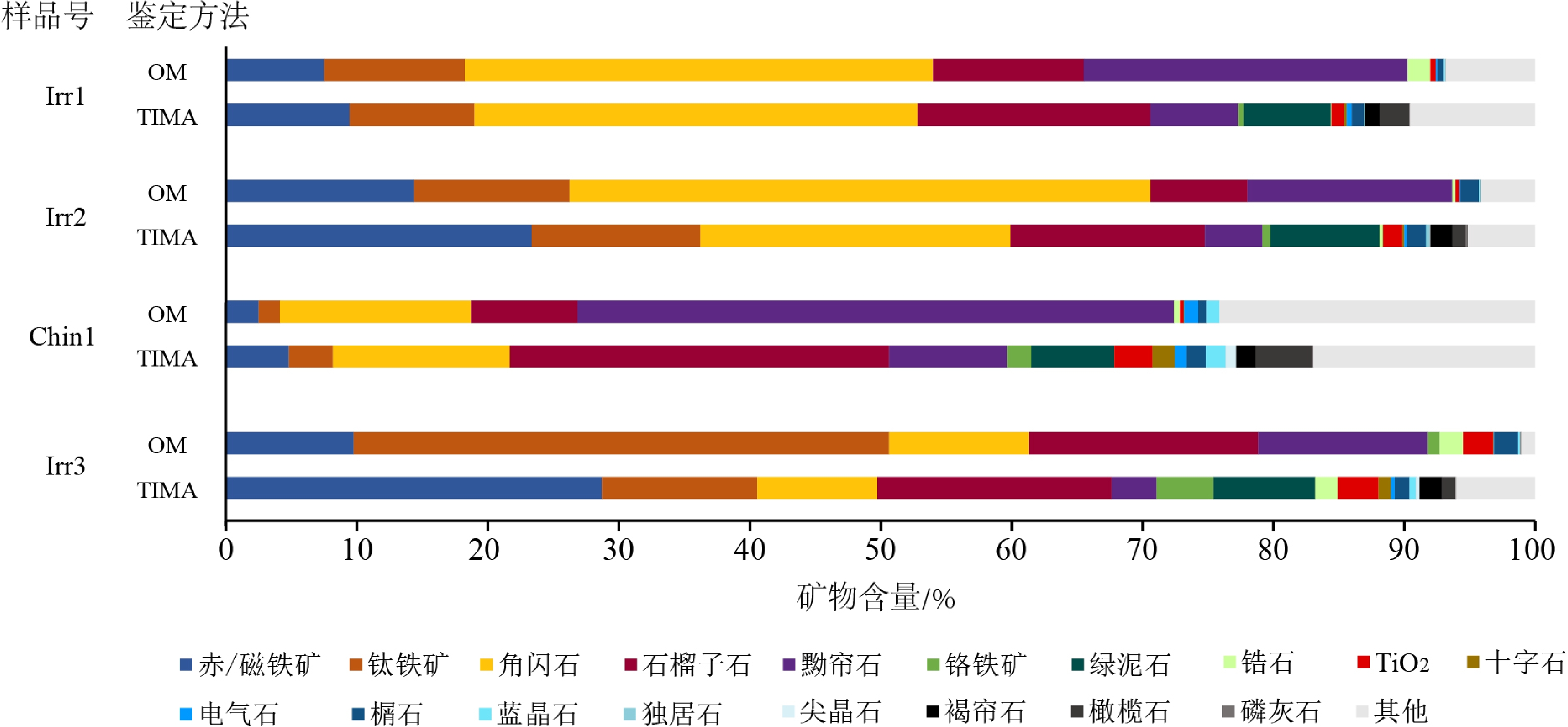
表 1 伊洛瓦底江沉积物样品信息
Table 1 Sample information of Irrawaddy sediments
样品号 纬度（N） 经度（E） 样品量/g 重矿物/mg 重矿物占比/% Irr1 23°51'25.03'' 96°13'49.00'' 900 21814 2.42 Irr2 22°26'42.23'' 96°1'00.23'' 1350 58694 4.35 Irr3 20°08'59.83'' 94°53'32.46'' 500 11747 2.35 Chin1 22°11'43.27'' 95°04'27.83'' 1100 5088 0.46 
下载: 导出CSV
表 2 TIMA及OM法测得的伊洛瓦底江沉积物重矿物含量
Table 2 Heavy mineral content of Irrawaddy River sediments by TIMA and OM methods
% 矿物名称
Irr1
Irr2
Chin1
Irr3TIMA OM TIMA OM TIMA OM TIMA OM 角闪石 / 35.75 / 44.35 / 14.60 / 10.68 普通角闪石 21.45 / 16.23 / 6.68 / 6.03 / 韭闪石 2.55 / 1.75 / 1.51 / 1.09 / 阳起石 9.88 / 5.70 / 5.32 / 2.04 / 赤铁矿 / 1.54 / 5.20 / 0.81 / 0.82 磁铁矿 / 5.98 / 9.19 / 1.70 / 8.94 赤/磁铁矿 9.48 / 23.36 / 4.79 / 28.74 / 钛铁矿 9.49 10.75 12.88 11.88 3.38 1.62 11.83 40.90 铬铁矿 0.42 — 0.61 — 1.84 △ 4.34 0.87 石榴子石 / 11.52 / 7.42 / 8.11 / 17.53 钙铝榴石 13.42 / 10.23 / 23.89 / 10.59 / 铁铝榴石 3.60 / 4.06 / 3.77 / 6.11 / 钙铁榴石 0.40 / 0.19 / 0.90 / 0.67 / 锰铝榴石 0.12 / 0.21 / 0.29 / 0.45 / 镁铝榴石 0.24 / 0.19 / 0.15 / 0.11 / 黝帘石 6.69 24.74 4.38 15.64 9.02 45.60 3.41 12.96 褐帘石 1.13 / 1.66 / 1.47 / 1.70 / 锆石 0.03 1.73 0.25 0.25 0.01 0.43 1.73 1.84 金红石 0.98 0.41 1.49 0.29 2.92 0.27 3.09 1.46 锐钛矿 / — / 0.02 / 0.02 / 0.57 白钛石 / — / — / 0.01 / 0.22 榍石 0.96 0.46 1.47 1.42 1.48 0.64 1.13 1.81 绿泥石 6.68 / 8.34 / 6.33 / 7.8 / 橄榄石 2.26 / 1.07 / 4.31 / 1.04 / 磷灰石 0.08 0.04 0.16 0.03 0.09 — 0.07 0.06 十字石 0.17 △ 0.15 — 1.74 0.05 0.96 0.05 电气石 0.39 0.14 0.22 0.07 0.88 1.06 0.29 0.05 蓝晶石 0.03 0.05 0.05 0.06 1.48 0.97 0.50 0.19 独居石 0.02 0.07 0.22 0.04 0.01 △ 0.00 △ 尖晶石 — △ 0.04 △ 0.83 * 0.25 △ 辉石 / △ / △ / △ / △ 白云母 0.59 / 0.28 / 0.50 / 0.14 / 黑云母 0.33 / 0.10 / 0.03 / 0.02 / 钡锰闪叶石 — / — / 0.08 / — / 刚玉 — / — / 0.07 / — / 方铁锰矿 — / — / 0.04 / — / 重晶石 — / — / — / 0.05 / 霓石 — / 0.03 / — / — / 其他 8.62 6.81 4.68 3.32 16.22 24.09 5.81 1.04 注：“—”表示矿物含量小于0.01%；“/”表示未发现该矿物；“△”代表0～500粒；“*”代表500～700粒；OM指光学显微镜法。
下载: 导出CSV
表 3 TIMA与OM法鉴定的伊洛瓦底江沉积物重矿物统一分类
Table 3 Unified classification of heavy mineral identification of Irrawaddy River sediments by TIMA and OM methods
% 矿物种类
Irr1
Irr2
Chin1
Irr3TIMA OM TIMA OM TIMA OM TIMA OM 稳定
重矿物赤/磁铁矿 9.48 7.52 23.36 14.39 4.79 2.51 28.74 9.76 钛铁矿 9.49 10.75 12.88 11.88 3.38 1.62 11.83 40.90 铬铁矿 0.42 — 0.61 — 1.84 △ 4.34 0.87 石榴子石 17.78 11.52 14.87 7.42 28.98 8.11 17.94 17.53 锆石 0.03 1.73 0.25 0.25 0.01 0.43 1.73 1.84 TiO2 0.98 0.41 1.49 0.31 2.92 0.30 3.09 2.25 十字石 0.17 △ 0.15 — 1.74 0.05 0.96 0.05 电气石 0.39 0.14 0.22 0.07 0.88 1.06 0.29 0.05 榍石 0.96 0.46 1.47 1.42 1.48 0.64 1.13 1.81 蓝晶石 0.03 0.05 0.05 0.06 1.48 0.97 0.50 0.19 独居石 0.02 0.07 0.22 0.04 0.01 △ 0.00 △ 尖晶石 — △ 0.04 △ 0.83 * 0.25 △ 不稳定
重矿物角闪石 33.88 35.75 23.68 44.35 13.51 14.60 9.16 10.68 黝帘石 6.69 24.74 4.38 15.64 9.02 45.60 3.41 12.96 褐帘石 1.13 / 1.66 / 1.47 / 1.70 / 绿泥石 6.68 / 8.34 / 6.33 / 7.8 / 橄榄石 2.26 / 1.07 / 4.31 / 1.04 / 磷灰石 0.08 0.04 0.16 0.03 0.09 — 0.07 0.06 注：“—”表示矿物含量小于0.01%；“/”表示未发现该矿物；“△”代表0～500粒；“*”代表500～700粒；OM指光学显微镜法。
下载: 导出CSV
-
[1] Clark M K, Schoenbohm L M, Royden L H, et al. Surface uplift, tectonics, and erosion of eastern Tibet from large-scale drainage patterns [J]. Tectonics, 2004, 23(1): TC1006.
[2] Brookfield M E. The evolution of the great river systems of southern Asia during the Cenozoic India-Asia collision: rivers draining southwards [J]. Geomorphology, 1998, 22(3-4): 285-312. doi: 10.1016/S0169-555X(97)00082-2
[3] 郑洪波, 王平, 何梦颖, 等. 长江东流水系建立的时限及其构造地貌意义[J]. 第四纪研究, 2013, 33(4):621-630 doi: 10.3969/j.issn.1001-7410.2013.04.01 ZHENG Hongbo, WANG Ping, HE Mengying, et al. Timing of the establishment of the east-flowing Yangtze River and tectonic-geomorphic implications [J]. Quaternary Sciences, 2013, 33(4): 621-630. doi: 10.3969/j.issn.1001-7410.2013.04.01
[4] Robinson R A J, Bird M I, Oo N W, et al. The irrawaddy river sediment flux to the Indian ocean: the original nineteenth‐century data revisited [J]. The Journal of Geology, 2007, 115(6): 629-640. doi: 10.1086/521607
[5] Robinson R A J, Brezina C A, Parrish R R, et al. Large rivers and orogens: The evolution of the Yarlung Tsangpo-Irrawaddy system and the eastern Himalayan syntaxis [J]. Gondwana Research, 2014, 26(1): 112-121. doi: 10.1016/j.gr.2013.07.002
[6] Liang Y H, Chung S L, Liu D Y, et al. Detrital zircon evidence from Burma for reorganization of the eastern Himalayan river system [J]. American Journal of Science, 2008, 308(4): 618-638. doi: 10.2475/04.2008.08
[7] Licht A, Reisberg L, France-Lanord C, et al. Cenozoic evolution of the central Myanmar drainage system: insights from sediment provenance in the Minbu Sub-Basin [J]. Basin Research, 2016, 28(2): 237-251. doi: 10.1111/bre.12108
[8] Zhang P, Najman Y, Mei L F, et al. Palaeodrainage evolution of the large rivers of East Asia, and Himalayan-Tibet tectonics [J]. Earth-Science Reviews, 2019, 192: 601-630. doi: 10.1016/j.earscirev.2019.02.003
[9] Licht A, France-Lanord C, Reisberg L, et al. A palaeo Tibet-Myanmar connection? Reconstructing the Late Eocene drainage system of central Myanmar using a multi-proxy approach [J]. Journal of the Geological Society, 2013, 170(6): 929-939. doi: 10.1144/jgs2012-126
[10] Licht A, Dupont-Nivet G, Win Z, et al. Paleogene evolution of the Burmese forearc basin and implications for the history of India-Asia convergence [J]. GSA Bulletin, 2019, 131(5-6): 730-748. doi: 10.1130/B35002.1
[11] Van Hoang L, Wu F Y, Clift P D, et al. Evaluating the evolution of the Red River system based on in situ U-Pb dating and Hf isotope analysis of zircons [J]. Geochemistry, Geophysics, Geosystems, 2009, 10(11): Q11008.
[12] Garzanti E, Wang J G, Vezzoli G, et al. Tracing provenance and sediment fluxes in the Irrawaddy River basin (Myanmar) [J]. Chemical Geology, 2016, 440: 73-90. doi: 10.1016/j.chemgeo.2016.06.010
[13] 杨仁超, 李进步, 樊爱萍, 等. 陆源沉积岩物源分析研究进展与发展趋势[J]. 沉积学报, 2013, 31(1):99-107 YANG Renchao, LI Jinbu, FAN Aiping, et al. Research progress and development tendency of provenance analysis on terrigenous sedimentary rocks [J]. Acta Sedimentologica Sinica, 2013, 31(1): 99-107.
[14] Pettijohn F J, Potter P E, Siever R. Sand and Sandstone[M]. New York: Springer, 1972.
[15] Mange M A, Maurer H F W. Heavy Minerals in Colour[M]. London: Chapman and Hall, 1992.
[16] Morton A C, Hallsworth C R. Processes controlling the composition of heavy mineral assemblages in sandstones [J]. Sedimentary Geology, 1999, 124(1-4): 3-29. doi: 10.1016/S0037-0738(98)00118-3
[17] Morton A C, Hallsworth C. Identifying provenance-specific features of detrital heavy mineral assemblages in sandstones [J]. Sedimentary Geology, 1994, 90(3-4): 241-256. doi: 10.1016/0037-0738(94)90041-8
[18] Zuffa G G, Serra F. Chapter 9 effects of hydrothermal fluids on the heavy mineral assemblage of a late pleistocene succession deposited in an oceanic ridge valley (Escanaba trough, Juan De Fuca Plate) [J]. Developments in Sedimentology, 2007, 58: 263-276.
[19] Sylvester P. Quantitative Mineralogy and Microanalysis of Sediments and Sedimentary Rocks[M]. St. John’s NL: Mineralogical Association of Canada, 2012: 25-26.
[20] 任迎新, 朱宝华. 重砂矿物分选及鉴定[M]. 武汉: 中国地质大学出版社, 1987. ZHU Yingxin, ZHU Baohua. Heavy Placer Mineral Separation and Identification[M]. Wuhan: China University of Geosciences, 1987.
[21] Sylvester P J. Use of the Mineral Liberation Analyzer (MLA) for Mineralogical Studies of Sediments and Sedimentary Rocks[M]. St. John’s NL: Mineralogical Association of Canada, 2012: 1-16.
[22] Hrstka T, Gottlieb P, Skála R, et al. Automated mineralogy and petrology - applications of TESCAN Integrated Mineral Analyzer (TIMA) [J]. Journal of Geosciences, 2018, 63(1): 47-63.
[23] 陈倩, 宋文磊, 杨金昆, 等. 矿物自动定量分析系统的基本原理及其在岩矿研究中的应用: 以捷克泰思肯公司TIMA为例[J]. 矿床地质, 2021, 40(2):345-368 CHEN Qian, SONG Wenlei, YANG Jinkun, et al. Principle of automated mineral quantitative analysis system and its application in petrology and mineralogy: An example from TESCAN TIMA [J]. Mineral Deposits, 2021, 40(2): 345-368.
[24] Dunkl I, Von Eynatten H, Andò S, et al. Comparability of heavy mineral data – The first interlaboratory round robin test [J]. Earth-Science Reviews, 2020, 211: 103210. doi: 10.1016/j.earscirev.2020.103210
[25] 崔颖颖, 周亚利, 陈国祥, 等. 毛乌素沙地样品扫描电镜的矿物定量分析[J]. 干旱区地理, 2020, 43(6):1505-1513 CUI Yingying, ZHOU Yali, CHEN Guoxiang, et al. Mineral quantitative analysis of Mu Us Sandy Land with QEMSCAN [J]. Arid Land Geography, 2020, 43(6): 1505-1513.
[26] 刘冬英, 陈玺, 黄燕, 等. 缅甸伊洛瓦底江流域水文资料初步复核评价[J]. 人民长江, 2018, 49(22):112-117 LIU Dongying, CHEN Xi, HUANG Yan, et al. Preliminary recheck and evaluation of hydrological data in Irrawaddy River Basin, Myanmar [J]. Yangtze River, 2018, 49(22): 112-117.
[27] Furuichi T, Win Z, Wasson R J. Discharge and suspended sediment transport in the Ayeyarwady River, Myanmar: centennial and decadal changes [J]. Hydrological Processes, 2010, 23(11): 1631-1641.
[28] Mitchell A H G, Htay M T, Htun K M, et al. Rock relationships in the Mogok metamorphic belt, Tatkon to Mandalay, central Myanmar [J]. Journal of Asian Earth Sciences, 2007, 29(5-6): 891-910. doi: 10.1016/j.jseaes.2006.05.009
[29] 刘锋, 赵越, 宋立才, 等. 伊洛瓦底江上游水系形成时代研究: 以滇西龙川江为例[J]. 中国地质, 2015, 42(1):199-206 doi: 10.3969/j.issn.1000-3657.2015.01.016 LIU Feng, ZHAO Yue, SONG Licai, et al. Time of the upper Irrawaddy streams: A case study of the Longchuan River, western Yunnan [J]. Geology in China, 2015, 42(1): 199-206. doi: 10.3969/j.issn.1000-3657.2015.01.016
[30] Chapman H, Bickle M, Thaw S H, et al. Chemical fluxes from time series sampling of the Irrawaddy and Salween Rivers, Myanmar [J]. Chemical Geology, 2015, 401: 15-27. doi: 10.1016/j.chemgeo.2015.02.012
[31] Zin W W, Nestmann F, Ihringer J. Flood forecasting using FGM model in Chindwin river basin [J]. Malaysian Journal of Civil Engineering, 2009, 21(2): 135-151.
[32] UNDGSE. Mineral exploration in selected areas, Burma[R]. New York: United Nations, 1979: 86.
[33] UNDGSE. Geological mapping and geochemical exploration in Mansi-Manhton, Indaw-Tigyaing, Kyindwe-Longyi, Patchaung-Yane and Yezin areas, Burma[R]. New York: United Nations, 1979: 13.
[34] Searle M P, Noble S R, Cottle J M, et al. Tectonic evolution of the Mogok metamorphic belt, Burma (Myanmar) constrained by U-Th-Pb dating of metamorphic and magmatic rocks [J]. Tectonics, 2007, 26(3): TC3014.
[35] Bender F. Geology of Burma[M]. Berlin: Gebrüder Borntraeger, 1983.
[36] Mitchell A H G. Cretaceous-Cenozoic tectonic events in the western Myanmar(Burma)-Assam region [J]. Journal of the Geological Society, 1993, 150(6): 1089-1102. doi: 10.1144/gsjgs.150.6.1089
[37] Thein M L. The lower paleozoic stratigraphy of western part of the Southern Shan State, Burma [J]. Bulletin of the Geological Society of Malaysia, 1973, 6: 143-163. doi: 10.7186/bgsm06197310
[38] Mitchell A, Chung S L, Oo T, et al. Zircon U–Pb ages in Myanmar: Magmatic–metamorphic events and the closure of a neo-Tethys ocean? [J]. Journal of Asian Earth Sciences, 2012, 56: 1-23. doi: 10.1016/j.jseaes.2012.04.019
[39] Brunnschweiler R O. On the geology of the Indoburman ranges [J]. Journal of the Geological Society of Australia, 1966, 13(1): 137-194. doi: 10.1080/00167616608728608
[40] Acharyya S K, Ray K K, Sengupta S. Tectonics of the ophiolite belt from Naga Hills and Andaman Islands, India [J]. Proceedings of the Indian Academy of Sciences - Earth and Planetary Sciences, 1990, 99(2): 187.
[41] Acharyya S K. Indo-Burma Range: a belt of accreted microcontinents, ophiolites and Mesozoic–Paleogene flyschoid sediments [J]. International Journal of Earth Sciences, 2015, 104(5): 1235-1251. doi: 10.1007/s00531-015-1154-6
[42] 和钟铧, 刘招君, 郭巍. 柴达木盆地北缘大煤沟剖面重矿物分析及其地质意义[J]. 世界地质, 2001, 20(3):279-284,312 doi: 10.3969/j.issn.1004-5589.2001.03.012 HE Zhonghua, LIU Zhaojun, GUO Wei. The heavy mineral analysis and its geological significance of Dameigou section in northern Caidam Basin [J]. World Geology, 2001, 20(3): 279-284,312. doi: 10.3969/j.issn.1004-5589.2001.03.012
[43] Mitchell A. Mogok metamorphic belt[M]//Mitchell A. Geological Belts, Plate Boundaries, and Mineral Deposits in Myanmar. Barcelona: Elsevier, 2018: 201-251.
[44] Mitchell A. Kumon range[M]//Mitchell A. Geological Belts, Plate Boundaries, and Mineral Deposits in Myanmar. Barcelona: Elsevier, 2018: 461-465.
[45] Enami M, Ko Z W, Win A, et al. Eclogite from the Kumon range, Myanmar: Petrology and tectonic implications [J]. Gondwana Research, 2012, 21(2-3): 548-558. doi: 10.1016/j.gr.2011.07.018
[46] Mitchell A. Popa-loimye magmatic arc[M]//Mitchell A. Geological Belts, Plate Boundaries, and Mineral Deposits in Myanmar. Barcelona: Elsevier, 2018: 277-323.
[47] 吴良士. 缅甸区域成矿地质特征及其矿产资源(一)[J]. 矿床地质, 2011, 30(1):176 doi: 10.3969/j.issn.0258-7106.2011.01.016 WU Liangshi. Regional metallogenic geological characteristics and mineral resources in Myanmar [J]. Mineral Deposits, 2011, 30(1): 176. doi: 10.3969/j.issn.0258-7106.2011.01.016
[48] Amidon W H, Burbank D W, Gehrels G E. Construction of detrital mineral populations: insights from mixing of U-Pb zircon ages in Himalayan rivers [J]. Basin Research, 2005, 17(4): 463-485. doi: 10.1111/j.1365-2117.2005.00279.x
[49] Xu C, Kynický J, Tao R B, et al. Recovery of an oxidized majorite inclusion from Earth's deep asthenosphere [J]. Science Advances, 2017, 3(4): e1601589. doi: 10.1126/sciadv.1601589
[50] 李胜荣, 许虹, 申俊峰, 等. 结晶学与矿物学[M]. 北京: 地质出版社, 2008. LI Shengrong, XU Hong, SHEN Junfeng, et al. Crystallography and Mineralogy[M]. Beijing: Geological Publishing House, 2008.
[51] Morton A C. Influences of provenance and diagenesis on detrital garnet suites in the Paleocene Forties sandstone, central North Sea [J]. Journal of Sedimentary Research, 1987, 57(6): 1027-1032.
-
期刊类型引用(3)
1. 刘景良，苏杰龙，戴逸宸，李宇祖，黄永，郑文婷. 基于滑动窗宽优化的LMSSGST识别非平稳信号瞬时频率. 振动与冲击. 2024(19): 183-193 . 
百度学术
2. 李雪英，王鑫. 二阶同步提取变换的沉积旋回界面定位与追踪识别. 黑龙江科技大学学报. 2023(04): 614-621 . 百度学术
3. 吴耀乐，邓敏. 基于残差卷积神经网络的随机噪声压制网络的海洋地震资料去噪技术研究. 中国石油和化工标准与质量. 2022(22): 175-177 . 百度学术
其他类型引用(4)
计量
- 文章访问数: 1635
- HTML全文浏览量: 419
- PDF下载量: 63
- 被引次数: 7
